Tangent
Definition
f(x)=tan(x)≡sin(x)cos(x).
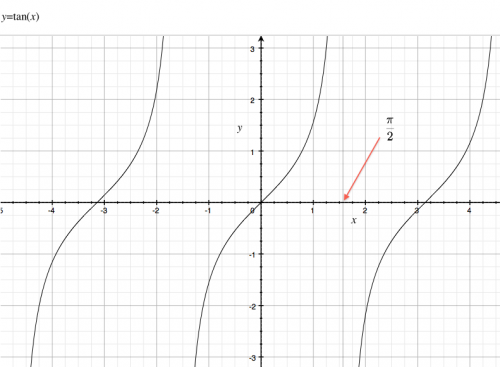
Graph
Properties
- The function tan is periodic with period π, not 2π like sin and cos.
- The tan function has asymptotes at all values of x for which the denominator (cos) goes to zero.
- The locations of the asymptotes are x=π2,−π2,π2,3π2,….
- At those values, tan approaches ∞ from the left, and −∞ from the right.
- Value at 0: tan(0)=01=0 because sin(0)=0.
- The angle x=π4 is special since both sin and cos are equal
and we get:
\[
\tan\left(\frac{\pi}{4} \right)
= \frac{ \sin\left(\frac{\pi}{4}\right) }{ \cos\left(\frac{\pi}{4}\right) }
= \frac{ \frac{\sqrt{2}}{2} }{ \frac{\sqrt{2}}{2} }
= 1.
\]