The page you are reading is part of a draft (v2.0) of the "No bullshit guide to math and physics."
The text has since gone through many edits and is now available in print and electronic format. The current edition of the book is v4.0, which is a substantial improvement in terms of content and language (I hired a professional editor) from the draft version.
I'm leaving the old wiki content up for the time being, but I highly engourage you to check out the finished book. You can check out an extended preview here (PDF, 106 pages, 5MB).
Muvement harmonique simple
Cette loi dit que les causes de l'acceleration c'est les forces sur un objet.
Concepts
- A: Amplitude du mouvement
- t: temps
- x(t): position de l'objet
- ω: fréquence angulaire,
- ϕ: constante phase
- (ωt+ϕ)=θ: phase, l'argument de la fonction sin
- T: période (combien de temps avant que le mouvement se répète) [s]
- f: fréquence f=1/T=ω2π [Hz] = [1/s]
il faut aussi essayer de vous rappeler le concept de derivee d'une fonction
Formules
Le mouvement d'un objet en “mouvement harmonique” a la forme de
x(t)=Asin(ωt+ϕ)
La commencencez pas a voir peur la. C'est vraiment simple. On va étudier la fonction sin un peu, apres ca on va utiliser x(t) et sa dérivée dx(t)dt=v(t) la vitesse
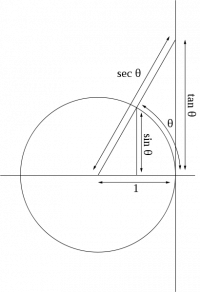
fonction sin(x)
f(x)=sin(x) et f(x)=cos(x) sont deux fonctions c'est a dire prennent un argument qui dans ce case est un angle θ Apartir de maintenant on va parler de θ comme ca on sait que c'est un angle pas une longeur
θ=0 correspond a l'axe des x
θ=π correspond a la direction −x
θ=C est une direction perpendiculaire a l'axe des x (aussi dite orthogonale). Pensez a votre coin de chambre. θ=π/2 c'est θ=90∘. Angle droit.
θ=π4: c'est quelque part au milieu entre 0 et π2, et ca correspond a l'angle 45∘ en degrés.
sin et cos ont rapport avec un triangle et un cercle
ces fonctions sont pour convertir du cercle au triangle
sin(0)=0
sin(π/2)=1
en fait cos c'est tres similaire mais completement oppose
Derivation
mais commencons par le debut
F=ma
Fxressort=−kx
a=dvdt plus precisememt a(t)=dv(t)dt
F=ma
−kx=ma −kx(t)=md2x(t)dt2
md2xdt2+kx(t)=0
d2xdt2+kmx(t)=0
x(t)=Asin(√kmt+ϕ)
ω=√km
T=2πω
Equation generale
x(t)=Asin(ωt+ϕ)
v(t)=Aωcos(ωt+ϕ)
a(t)=−Aω2sin(ωt+ϕ)
Energy
Potential energy is :
U=12kx2=12kA2sin2(ωt+ϕ)
Cinematic energy is :
K=12mv2=12mω2A2cos2(ωt+ϕ)
Total energy:
Etotal=Umax=12kA2=Kmax=12mv2
Total energy:
ET=U+K
Conservation of energy says:
Ei=Ef
so in this case
Ui+Ki=Uf+Kf
Examples
example 1
voici un exemple.
example 2
voici un autre !
Discussion
Des fois quand il n'y a pas d'acceleration ax=0 ca peut nous permettre…