The page you are reading is part of a draft (v2.0) of the "No bullshit guide to math and physics."
The text has since gone through many edits and is now available in print and electronic format. The current edition of the book is v4.0, which is a substantial improvement in terms of content and language (I hired a professional editor) from the draft version.
I'm leaving the old wiki content up for the time being, but I highly engourage you to check out the finished book. You can check out an extended preview here (PDF, 106 pages, 5MB).
Light rays
In this section we will study how light rays get reflected off the surfaces of objects and what happens when light rays reach the boundary between two different materials.
Definitions
The speed of light depends on the material where it travels:
- vx: the speed of light in material x.
- nx: the diffraction index of material x,
which tells you how much slower light is in that material.
$v_x=c/n_x$.
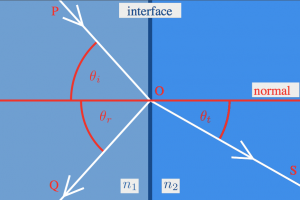
When an incoming ray of light comes to the surface of a transparent object, part of it will be reflected and part of it will be transmitted. We measure all angles with respect to the normal, which is the direction perpendicular to the interface.
- θi: The incoming or incidence angle.
- θr: The reflection angle.
- θt: The transmission angle:
the angle of the light that goes into the object.
Formulas
Reflection
Light that hits a reflective surface will bounce back exactly at the same angle as it came in on: θi=θr.
Refraction
The transmission angle of light when it goes into a material with different refractive index can be calculated from Snell's law: nisinθi=ntsinθt.
Total internal refraction
Light coming in from a medium with low refraction index into a medium with high refraction index gets refracted towards the normal. If the light travels in the opposite direction (from high n, to low n), then it will get deflected away from the normal. In the latter case, an interesting phenomenon called total internal refraction occurs, whereby light rays incident at sufficiently large angles with the normal get trapped inside the material. The angle at which this phenomenon starts to kick in is called the critical angle θcrit.
Consider a light ray inside a material of refractive indeed nx surrounded by a material with smaller refractive index ny, nx>ny. To make this more concrete, think of a trans-continental underground optical cable made of glass nx=1.7 surrounded by some plastic with ny=1.3. All light at an angle greater than: θcrit=sin−1(nynxsin(90∘)⏟=1)=sin−1(nynx)=sin−1(1.31.7)=49.88∘, will get reflected every time it reaches the surface of the optical cable. Thus, if you shine a laser pointer into one end of such a fibre-optical cable in California, 100% of that laser light will come out in Japan. Most high-capacity communication links around the world are based around this amazing property of light. In other words: no total internal refraction means no internet.
Examples
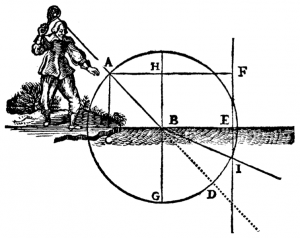
What is wrong in this picture?
Here is an illustration from one of René Descartes' books, which shows a man in funny pants with some sort of lantern which produces a light ray that goes into the water.
Q: What is wrong with the picture?
Hint: Recall that nair=1 and nwater=1.33, so ni<nt.
Hint 2: What should happen to the angles of the light ray?
A: Suppose that the line ¯AB, is at 45∘ angle, then after entering the water at B, the ray should be deflected towards the normal, i.e., it should pass somewhere between G and D. If you wanted to be precise and calculate the transmission angle then we would use: nisinθi=ntsinθt, filled in with the values for air and water 1sin(45∘)=1.33sin(θt), and solved for θt (the refracted angle) we would get: θt=sin−1(√22×1.33)=32.1∘. The mistake apparently is due to Descartes' printer who got confused and measured angles with respect to the surface of the water. Don't make that mistake: remember to always measure angles with respect to the normal. The correct drawing should have the light ray going at an angle of 32.1∘ with respect to the line ¯BG.
Explanations
Refraction
To understand refraction you need to imagine “wave fronts” perpendicular to the light rays. Because light comes in at an angle, one part of the wave front will be in material ni and the other will be in material nt. Suppose ni<nt, then the part of the wavefront in the nt material will move slower so angles of the wavefronts will change. The precise relationship between the angles will depend on the refractive indices of the two materials:
nisinθi=ntsinθt.
Total internal refraction
Whenever ni>nt, we reach a certain point where the formula: nisinθi=ntsinθt, brakes down. If the transmitted angle θt becomes greater than 90∘ (the critical transmission angle) it will not be transmitted at all. Instead, 100% of the light ray will get reflected back into the material.
To find the critical incident angle solve for θi in: nisinθi=ntsin90∘, θcrit=sin−1(ntni).
The summary of the “what happens when a light ray comes to a boundary”-story is as follows:
- If −θcrit<θi<θcrit, then some part of the light will be
transmitted at an angle θt and some part will be reflected at an angle θr=θi.
- If θi≥θcrit, then all the light will get reflected at an angle θr=θi.
Note that when going from a low n medium into a high n medium, there is no critical angle – there will always be some part of the light that is transmitted.