The page you are reading is part of a draft (v2.0) of the "No bullshit guide to math and physics."
The text has since gone through many edits and is now available in print and electronic format. The current edition of the book is v4.0, which is a substantial improvement in terms of content and language (I hired a professional editor) from the draft version.
I'm leaving the old wiki content up for the time being, but I highly engourage you to check out the finished book. You can check out an extended preview here (PDF, 106 pages, 5MB).
Sine
Contrary to what religious leaders might lead you to believe, there is nothing wrong with sin. At least not with the mathematical sin. The sinus, sine or sin function tells you the ratio of the lengths of two sides in a right angle triangle.
Definition
Let us not talk about triangles, angles and lengths of sides for now, but think of the sin function as a generic function of x: f(x)=sin(x).
Properties
- The sin function is odd, which the mathematical term for saying:
f(−x)=−f(x).
- The function is periodic, with period 2π, that is:
f(x)=f(x+2π).
- Relation to cos: sin2x+cos2x=1.
- Relation to csc: csc(x)≡1sinx (csc is read cosecant).
- The inverse function is sin−1(x)=arcsin(x), and is not
to be confused with (sin(x))−1=1sin(x)≡csc(x).
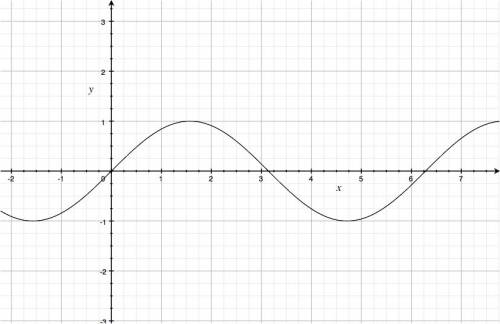
Graph
The function starts from zero sin(0)=0, then goes up to take on the value 1 at x=π4, then falls down until it crosses the x axis at x=π.
After π the function drops below the x axis and reaches its minimum value of −1 at x=3π2 only to come up again and repeat the 2π-long cycle starting from x=2π.
We have 0=sin(0)=sin(π)=sin(2π)=sin(3π)=⋯, in fact sin(x) has a root at each multiples of π.