The page you are reading is part of a draft (v2.0) of the "No bullshit guide to math and physics."
The text has since gone through many edits and is now available in print and electronic format. The current edition of the book is v4.0, which is a substantial improvement in terms of content and language (I hired a professional editor) from the draft version.
I'm leaving the old wiki content up for the time being, but I highly engourage you to check out the finished book. You can check out an extended preview here (PDF, 106 pages, 5MB).
Golden ratio
The golden ratio, usually denoted ϕ≈1.6180339887 is very important proportion in geometry, art, aesthetics, biology and mysticism. If you have a stick of length 1m and you wan to cut it into two pieces, say from 0 (the left) to x and from x to 1 (the right end). You have to pick the point x closer to the right end, such that the ratio of the lengths of the short piece and the long piece is the same as the ratio of the long piece and the whole stick. Mathematically, this means this: lremaining shortllong≡1−xx=x1m≡llonglwhole.
To see how the quadratic equation comes about, just multiply both sides by x to get: 1−x=x2, which after moving all the terms to one side becomes x2+x−1=0.
Using the quadratic formula we get the two solutions which are x1=−1+√52=1ϕ≈0.618030,x2=−1−√52=−ϕ≈−1.61803. The solution x2 is some negative number, so it cannot be the x we want – we wanted a ratio, i.e., 0≤x≤1. The golden ration then is ϕ=1x1=2√5−1=2√5−1√5+1√5+1=2(√5+1)5−1=√5+12.
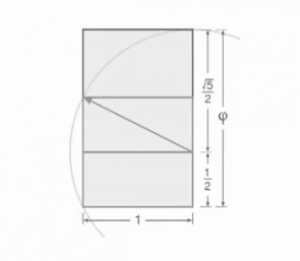
Geometry
Trigonometric functions
cos(π5)=cos36∘=√5+14=φ2
sin(π10)=sin18∘=√5−14=φ−12=12φ
Fibonacci connection
The Fibonacci sequence, is defined by the recurrence relation: Fn=Fn−1+Fn−2 with F1=1, and F2=1.
Binet's formula
The nth term in the Fibonacci sequence has a closed formed expression Fn=ϕn−(1−ϕ)n√5, where ϕ=1+√52 is the golden ratio.
in the limit of large n, this term will dominate the rate of growth of the Fibonacci sequence. Consider the ratio between Fn and Fn−1, i.e. at what rate is the sequence growing? FnFn−1=ϕn−(1−ϕ)nϕn−1−(1−ϕ)n−1
http://en.wikipedia.org/wiki/Fibonacci_number#Closed-form_expression
Five-pointed star
The golden ratio also appears in the ratios of two lengths in the pentagram, or five-pointed star. I am choosing not to discuss this here, because the five-pointed star is a symbol associated with satanism, freemasonry and communism, and I want nothing to do with any of these.
Electronic circuits
Links
[ The golden ratio in web design ]
http://www.pearsonified.com/2011/12/golden-ratio-typography.php